Formula for Ohm’s law of current and Voltage with graph

There are two basic laws of current electricity – one is Ohm’s law and another one is Kirchhoff’s law. Ohm’s law of current electricity gives the simplest relation between the electric current and voltage across a resistance. Kirchhoff’s laws tell us how to calculate electric current and voltage at a junction point or in a branch. I’ve explained Kirchhoff’s laws in another article. In this article, I’m going to discuss the statement, formula and other facts for ohm’s law of current and voltage. Also, the graphical plot and the applications will be discussed here.

Contents of this article:

• Statement of Ohm’s law
• Equation or formula for Ohm’s law
• Explanation of Ohm’s law
• Graph for ohm’s law
• Uses of Ohm’s Law
• Limitations of Ohm’s Law

Definition of Ohm’s law of Electricity

The definition refers to the statement. Ohm’s law of current electricity states that if the temperature and other physical conditions remain constant then the electric current through a conducting wire is directly proportional to the potential difference or the voltage applied across the wire and the proportional constant is equal to the inverse of the resistance of the wire.

Formula for Ohm’s law in Electricity

Let V is the applied voltage across a wire of resistance R and thereby the electric current flow through the wire is I. Then Ohm’s law tells that, \small {\color{Blue} I\propto V}

or, \small {\color{Blue} I=kV}, where, k is the proportional constant whose value is equal to the inverse of the resistance (R) of the wire. Then we get,

The formula of Ohm’s law as, \small {\color{Blue} I=\frac{1}{R}V}

or, \small {\color{Blue} V=IR}……………… (1)

Equation-(1) gives the mathematical equation for Ohm’s law.

Explanation of Ohm’s law

Ohm’s law gives the relation between current and voltage through a conductor. Equation-(1) includes the resistance of the conductor. If we apply a voltage of 10 volts across a conductor of resistance 10 ohms, then using I = V/R formula we get, the current through the conductor as I = 1 ampere. This is how one can find the current through the resistance or the conductor.

Looking at the equation you may think that the voltage is proportional to the current. Yes, one can say that, but this is not the actual concept. Because the voltage is an independent quantity which is applied externally and the current flows through the resistance only due to the applied voltage. Thus the current depends on the applied voltage, not the reverse case. That is why the correct concept is that the current is proportional to the voltage.

Current-Voltage (I-V) graph for a resistor

The current through the wire increases with the increase in voltage across it. One should use the equation \small {\color{Blue} I=\frac{1}{R}V} to draw the current versus voltage graph. Because voltage is the independent variable and the current is the dependable variable. So, we need to vary the voltage along X-axis and the current along Y-axis. Here, we get a Y = mX type equation with slope 1/R which implies a straight line passing through the origin.

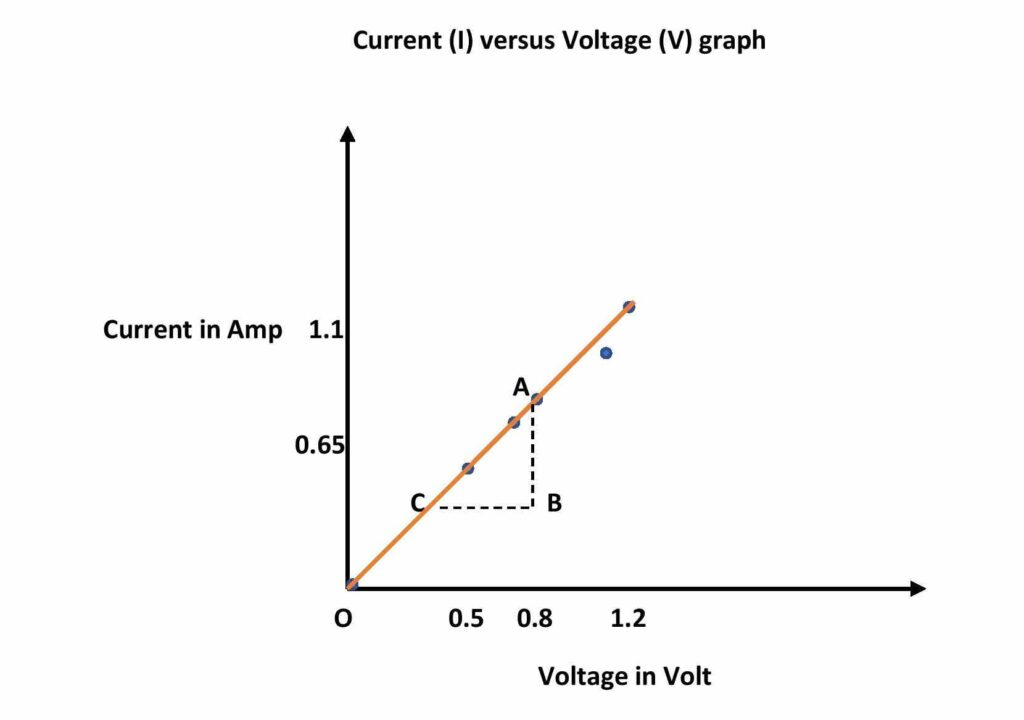
Then the current versus voltage graph in ohm’s law will give a straight line passing through the origin with the slope inverse of the resistance of the wire. The above diagram shows a current versus voltage graph with some experimental data for the verification of Ohm’s law practically. Here, the slope of the curve is AB/BC which is the inverse of the resistance of the conductor.

Uses of Ohm’s equation

Ohm’s law is used to find the current through a resistance when the resistance and the voltage are known. Actually, any of the three physical quantities (I, V and R) can be found if the other two are supplied. Also, one can give the definition of resistance of a conductor from Ohm’s law.

Let’s take an example problem to understand the thing mathematically.

The current through a conductor is 5 A when a 10 volt battery is connected across it. What will be the current through the conductor if the voltage applied across it is 12 volt?

When the voltage is V = 10 volts, the current through the conductor is I = 5 amps.

Then using the equation of Ohm’s law, the resistance of the conductor is, \small {\color{Blue} R=\frac{V}{I}} = \small {\color{Blue}\frac{10}{5}} = 2 ohms.

In the second case, the voltage is V = 12 volts and we got the resistance R = 2 ohms. Using the same equation, we get,

The current through the conductor is \small {\color{Blue} I=\frac{V}{R}} = \small {\color{Blue} I=\frac{12}{2}}= 6 amps.

This is how we can calculate anything among I, V and R by using Ohm’s equation.

Limitation of Ohm’s law

There are two major limitations of ohm’s law. These are –

• Ohm’s law is not applicable for non-linear circuit components like diodes, Transistors, etc. It is only applicable for linear circuit elements like resistors, capacitors, inductors, etc.
• Another limitation is that the resistance of the wire changes with the change in temperature which cause an uneven change of the current with the change in voltage. Therefore, we need to fix the temperature of the conductor.

Homework problems:

1. If 12 volt battery across a wire causes the 8 ampere current to flow through the wire then what is the resistance of the wire?
2. Find the ratio of current to voltage if the resistance of the conductor is 5 ohms.
3. How one can give the definition of resistance of a conductor by using the formula for Ohm’s law?

This is all from this article on Ohm’s law of current electricity that relates electric voltage, current and resistance. Hope you will be able to calculate these physical quantities from a given circuit. Read Kirchhoff’s laws to gather more knowledge on such calculations.

Thank you!

Related posts:

1. Verification of Ohm’s law experiment
2. Carbon resistor color code
3. Electric circuit components – Resistor, capacitor, Inductor, Diode and their uses.
4. Kirchhoff’s law of Current and Voltage – KCL and KVL
5. Series and parallel combination of resistors
6. Why do metals conduct electricity?