Biot-Savart Law of Magnetism and its formula

We have discussed the origin and definition of magnetic field. A bar magnet, a current-carrying wire and a moving charge are the origins of magnetic field. Then we learned to find the direction of magnetic field in another article. Now, how to find the magnitude or value of the magnetic field? Biot-Savart law of magnetostatics is a fundamental law that helps to find the magnitude of the magnetic field at a distance from a current carrying wire or conductor. In this article, I’m going to explain the Biot Savart law, its statement, formula, vector form, uses and limitations.

Contents in this article:

1. Statement
2. Formula
3. Vector form
4. Application
5. How to use the Biot Savart law to find the Magnetic field?
6. Limitation of Biot Savart law

Statement of Biot-Savart law of magnetism

Biot Savart law of magnetostatics states that the magnetic field at a small distance from an elementary portion of a current carrying wire is

directly proportional to the

1. current through the wire (I)
2. length of the elementary portion of the wire (dL)
3. sine of the angle between the direction of elementary length and the distance vector.

and inversely proportional to the square of the distance (r) of the target point from the elementary length.

Formula of Biot Savart law to find magnetic field

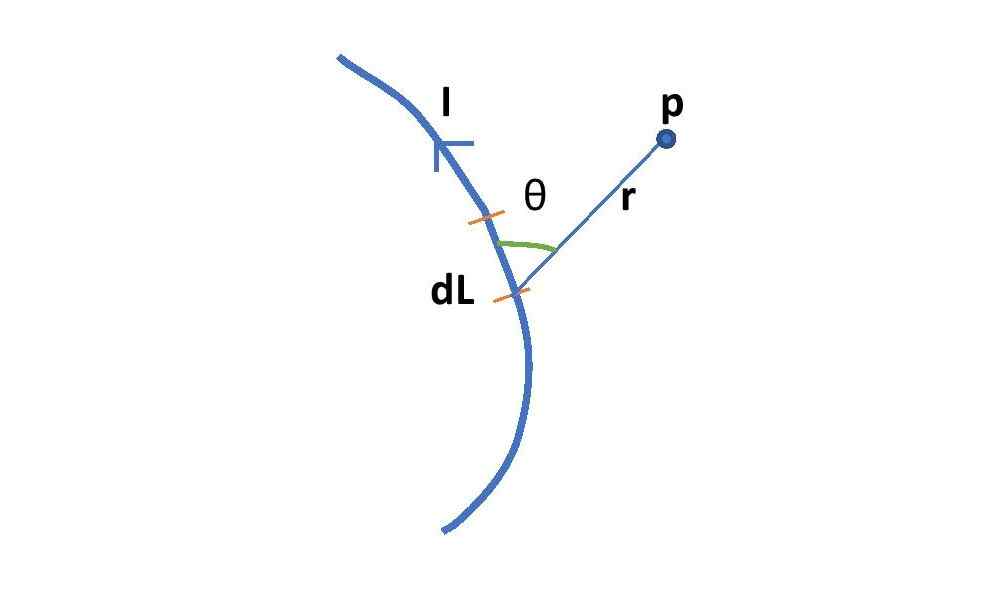
Let we want to find the magnetic field at a point P at r distance from an elementary length dL of a wire carrying a current I and the r makes an angle \small \theta with dL. Then according to the Biot-Savart law, the magnetic field (dB) at that point is,

1 \small {\color{Blue} dB\propto I}

2. \small {\color{Blue} dB\propto dL}

3. \small {\color{Blue} dB\propto sin\theta }

4. \small {\color{Blue} dB\propto \frac{1}{r^{2}}}

Now, taking all the variables together, we get, \small {\color{Blue} dB\propto \frac{IdLsin\theta }{r^{2}}}

or, \small {\color{Blue} dB=k\frac{IdLsin\theta }{r^{2}}} ………………….. (1)

Now, in SI system, the constant, \small k=\frac{\mu _{0}}{4\pi }. Where \mu _{0} is the permeability in free space.

Then, one can get the formula of Biot-Savart law as,

\small {\color{Blue} dB=\frac{\mu _{0}}{4\pi }\frac{IdLsin\theta }{r^{2}}} …………………… (2)

Vector form of Biot-Savart law

we got the mathematical form of Biot Savart law in equation-(2). Now one can write the vector form of Biot-Savart law as, \small {\color{Blue} d\vec{B}=\frac{\mu _{0}I}{4\pi }\frac{d\vec{L}\times \hat{r}}{r^{2}}} ………………. (3)

Again, \small \vec{r}=r\hat{r} then, \small \hat{r}=\frac{\vec{r}}{r}

so, one can re-write the vector form as, \small {\color{Blue} d\vec{B}=\frac{\mu _{0}I}{4\pi }\frac{d\vec{L}\times\vec{r}}{r^{3}}}………… (4)

Both of equation-(3) and (4) are the vector forms of Biot-Savart law.

Application of Biot-Savart law

Biot Savart law is used to find the magnetic field due to a current-carrying wire. One can use this law to find the

1. Magnetic field due to a current carrying straight wire.
2. Magnetic field at the center and at axial point of a current carrying circular wire.

Also, the direction of magnetic field can be found from the vector formula of this law by using Fleming’s Thumb rule or the Maxwell’s corkscrew rule.

How to use Biot Savart law to find the Magnetic field?

One can find magnetic field due to a current carrying wire by using the formula of Biot Savart law. To use this law we need to know the value of current through the wire, distance of target point where we want to find the magnetic field and the angle between the length and the distance vector. You need to familiar with the concepts of vector product too. While derivation, there may arise more than one variables. You need to convert those into single variable by using the relations between the variables. To understand this, one can check the procedure of finding the magnetic field due to a current carrying straight wire by using Biot Savart law.

In equation-(2), one can see that the magnetic field increases with the increase in current through the wire and decreases with the increase in distance from the wire. Also, the magnetic field depends on the medium. Magnetic permeability is the property of the medium on which the magnetic field depends. Again, if we take the wire of greater length and of same current then the strength of magnetic field will be greater at the same point.

Limitation of Biot Savart law

Biot Savart law cannot be used in any condition and for any conductors. There are certain limitations of this law.

1. Biot-Savart law is applicable when the current through the wire is steady. It cannot give the accurate result for changing current.
2. This law is not applicable for alternating current (AC) passing through the source wire.
3. Biot-Savart law is accurately applicable for infinitesimal current elements. It cannot be used for any type of current distribution or for any shaped conductor.

This is all from this article on how to find the magnetic field using Biot-Savart law of magnetism. If you have any doubt on this topic you can ask me in the comment section.

Thank You!

Related Posts:

1. Magnetic field due to a current carrying straight wire
2. Magnetic field due to a circular coil
3. Ampere’s Circuital Law